As technology advances, the limitations of conventional electronic computers are becoming increasingly apparent, especially when tackling complex computational challenges. NP-complete problems, which grow exponentially with size, represent some of the toughest puzzles in computer science. These issues have significant implications across various fields, including biomedicine, transportation, and manufacturing. In the quest for more effective solutions, researchers are exploring alternatives to traditional computing methods, with optical computing emerging as a promising avenue.
As reported in Advanced Photonics, a team from Shanghai Jiao Tong University has made strides in this area by developing a reconfigurable three-dimensional integrated photonic processor specifically designed to tackle the subset sum problem (SSP), a classic NP-complete problem.
Using an innovative technique called femtosecond laser direct writing, the researchers constructed a photonic chip composed of 1,449 standardized optical components. This technology allows for rapid prototyping and offers greater design flexibility, crucial for addressing the complexities of the SSP.
The subset sum problem involves determining whether a specific subset of numbers can sum to a given target. By mapping this problem onto their photonic processor, the researchers can encode the behavior of light to perform calculations. The processor operates by allowing photons in a light beam to explore all possible paths simultaneously, providing answers in parallel. This design not only speeds up the computation but also maintains high accuracy—demonstrated by the processor’s ability to solve different instances of the SSP with 100 percent reliability.
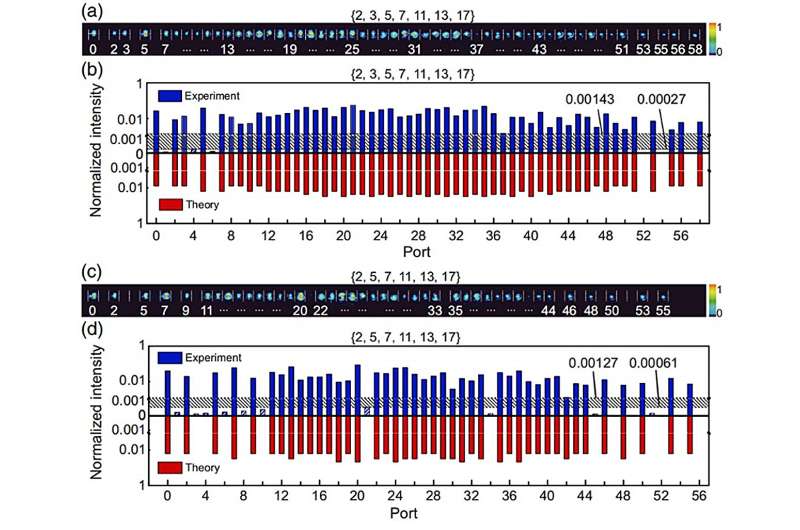
The potential applications for this technology extend beyond the subset sum problem. The reconfigurable nature of the processor could be adapted for tasks such as optical neural networks and photonic quantum computing, indicating a versatile future for photonic systems. Remarkably, this new processor has shown improved performance compared to existing electronic counterparts, particularly in computing time and efficiency as problem size increases.
This development marks a significant step toward harnessing the capabilities of light for practical computing, providing a pathway to address computationally demanding problems at a larger scale. As researchers continue to explore optical computing, this breakthrough could reshape how we approach complex challenges across various scientific and industrial domains.
More information:
Xiao-Yun Xu et al, Reconfigurable integrated photonic processor for NP-complete problems, Advanced Photonics (2024). DOI: 10.1117/1.AP.6.5.056011
Citation:
Solving computationally hard problems with 3D integrated photonics (2024, October 23)
retrieved 24 October 2024
from https://techxplore.com/news/2024-10-hard-problems-3d-photonics.html
This document is subject to copyright. Apart from any fair dealing for the purpose of private study or research, no
part may be reproduced without the written permission. The content is provided for information purposes only.

